is the answer of 7 is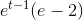 ?
?
 Sushovan Halder no.
Sushovan Halder no.
1) Prove that ∫x3√2ax-x2dx=7Ï€a5/8.
2) Integrate: [ x4/(1-x4)] *cos-1(2x/(1+x2).
Upper limit=1/√3;Lower limit=-1/√3.
3) Integrate::: 2-x2/[(1+x)√1-x2].Upper limit=1;Lower limit=0.
4) Integrate:::: log(1+tanx) from 0 to π/2.
5) Integrate::: (√3cos2x-1)/cosx from 0 to Ï€/6.
6) integrate:::: (cos9x)/(cos3x + sin3x) frm 0 to π/2.
7) If f(y)=ey; g(y)=y, y>0 and F(t)=∫f(t-y)g(y)dy from 0 to 1 then F(t)=?
8) Integrate:: [x]f'(x) from 0 to a ,where [.] is the G.I.F. function.
9) Integrate:::::: x4(1-x)4/(1+x2) from 0 to 1.
\hspace{-16}$For $\bf{(4)::}$ Given $\bf{\int_{0}^{\frac{\pi}{2}}\ln(1+\tan \phi)d\phi}$\\\\\\ Let $\bf{\tan \phi = x\;,}$ Then $\bf{\sec^2 \phi d\phi = dx\Rightarrow d\phi = \frac{1}{1+x^2}dx}$\\\\\\ And Changing Limit............, We Get\\\\\\ So Integral is $\bf{\int_{0}^{\infty}\frac{\ln(1+x)}{1+x^2}dx = \int_{0}^{1}\frac{\ln(1+x)}{1+x^2}dx+\int_{1}^{\infty}\frac{\ln(1+x)}{1+x^2}dx}$\\\\\\ Now Let $\bf{I=\int_{0}^{1}\frac{\ln(1+x)}{1+x^2}dx}$ and $\bf{J=\int_{1}^{\infty}\frac{\ln(1+x)}{1+x^2}dx}$\\\\\\ Now For Calculation of $\bf{I=\int_{0}^{1}\frac{\ln(1+x)}{1+x^2}dx}$\\\\\\ Put $\bf{x=\frac{1-t}{1+t}}$ and $\bf{dx=-\frac{2}{(1+t)^2}dt}$ and Changing Limit\\\\\\ So $\bf{I = \int_{0}^{1}\frac{\ln\left(\frac{2}{1+t}\right)}{1+t^2}dt = \ln(2)\cdot \int_{0}^{1}\frac{1}{1+t^2}dt-\int_{0}^{1}\frac{\ln(1+t)}{1+t^2}dt}$\\\\\\ So $\bf{I = \ln(2)\cdot \frac{\pi}{4}-I\Rightarrow I=\frac{\pi}{8}\cdot \ln(2)}$\\\\\\
\hspace{-18}$Now For Calculation of $\bf{J=\int_{1}^{\infty}\frac{\ln(1+x)}{1+x^2}dx}$\\\\\\ Put $\bf{x=\frac{1}{y}}$ and $\bf{dx = -\frac{1}{y^2}dy}$ and Changing Limit.....\\\\\\ So $\bf{J=\int_{0}^{1}\frac{\ln\left(\frac{1+y}{y}\right)}{1+y^2}dy = \int_{0}^{1}\frac{\ln(1+y)}{1+y^2}dx-\int_{0}^{1}\frac{\ln(y)}{1+y^2}dy}$\\\\\\ So $\bf{J=I+\mathbb{G}\;\;,}$ Where $\bf{\mathbb{G}=-\int_{0}^{1}\frac{\ln(y)}{1+y^2}dy=Catalan\; Constant.}$\\\\\\ So $\bf{\int_{0}^{\frac{\pi}{2}}\ln\left(1+\tan \phi\right)d\phi = I+J=I+I+\mathbb{G}}$\\\\\\ So $\bf{\int_{0}^{\frac{\pi}{2}}\ln\left(1+\tan \phi\right)d\phi = 2\cdot \frac{\pi}{8}\cdot \ln(2)+\mathbb{G}=\frac{\pi}{4}\ln(2)+\mathbb{G}}$\\\\\\
9)
Just divide the numerator by (1+x2) and proceed