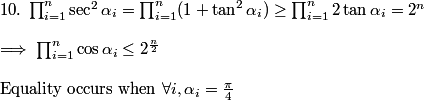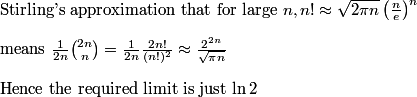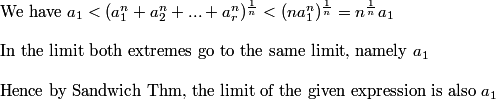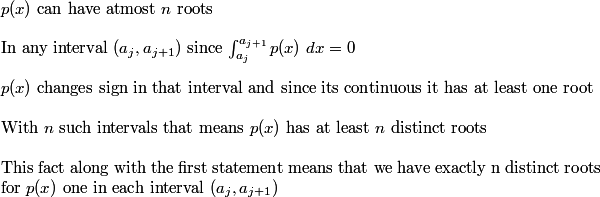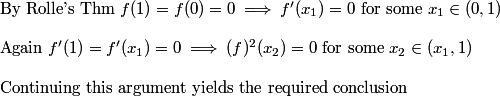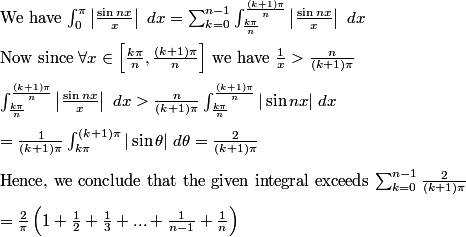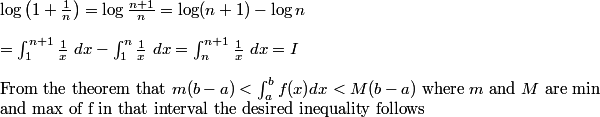\hspace{-16}(6)\;\; $Let $\bf{I_{2k+1} = \int_{0}^{\frac{\pi}{2}}\frac{\sin (2k+1)x}{\sin x}dx.........(1)}$\\\\\\ Replace $\bf{k\rightarrow (k+1)}$\\\\\\ $\bf{I_{2k+3} = \int_{0}^{\frac{\pi}{2}}\frac{\sin (2k+3)x}{\sin x}dx................(2)}$\\\\\\ So $\bf{I_{2k+3}-I_{2k+1} = \int_{0}^{\frac{\pi}{2}}\frac{\sin (2k+3)x-\sin (2k+1)x}{\sin x}dx}$\\\\\\ So $\bf{I_{2k+3}-I_{2k+1} = \int_{0}^{\frac{\pi}{2}}\frac{2\cos (2k+2)x\cdot \sin x}{\sin x}dx =0}$\\\\\\ So $\bf{I_{2k+3} = I_{2k+1}.}$\\\\\\ Now Replace $\bf{k\rightarrow (k-1)}$\\\\\\ $\bf{I_{2k+1} = I_{2k-1}}$\\\\\\ Using Recursively, We Get\\\\\\ $\bf{I_{2k+3} = I_{2k+1} = I_{2k-1}=I_{2k-3}=........=I_{3}}$\\\\\\ Now $\bf{I_{1} = \int_{0}^{\pi}\frac{\sin (2\cdot 1+1)}{\sin x}dx = \int_{0}^{\frac{\pi}{2}}\frac{3\sin x -4\sin ^3 x}{\sin x}=\frac{\pi}{2}}$\\\\\\
\hspace{-16}$So $\bf{I_{2k+3} = I_{2k+1} = I_{2k-1}=I_{2k-3}=........=I_{3} =\frac{\pi}{2}}$\\\\\\So $\bf{I_{2k+1}=\int_{0}^{\frac{\pi}{2}}\frac{\sin (2k+1)x}{\sin x}dx = \frac{\pi}{2}}$